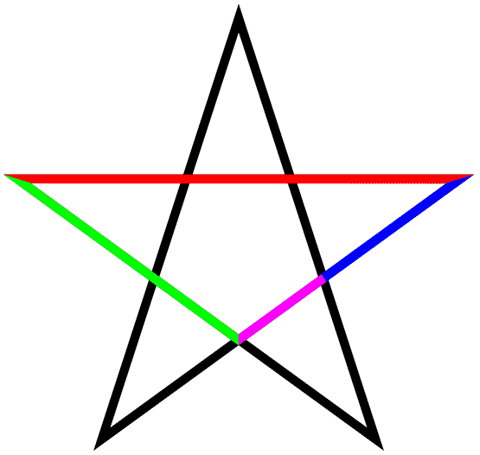
Above is a pentagram colored to distinguish its line segments of different lengths. The four lengths — defined at the intersection of edges — are in golden ratio to one another. (The red is 1.618 times the length of the green; the green is 1.618 times the length of the blue; the blue is 1.618 times the length of the magenta.)
Also, the ratio of the length of the shorter segment to the segment bounded by the 2 intersecting edges (a side of the pentagon in the pentagram’s center) is φ, as the four-color illustration shows.
The pentagram includes ten isosceles triangles: five acute and five obtuse isosceles triangles. In all of them, the ratio of the longer side to the shorter side is φ. The acute triangles are golden triangles. The obtuse isosceles triangles are golden gnomon.












Comments on this entry are closed.